Generated on Mon Aug 25 11:35:46 2008 for Gecode by doxygen 1.5.6

Simple relation constraints over integer variables
[Using finite domain integers]


Functions

void Gecode::rel (Space *home, const IntVarArgs &x, IntRelType r, IntVar y, IntConLevel icl=ICL_DEF, PropKind pk=PK_DEF)
 Post propagators for 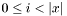$ x_i \sim_r y $ for all $0\leq i<|x|$.
void Gecode::rel (Space *home, IntVar x, IntRelType r, int c, IntConLevel icl=ICL_DEF, PropKind pk=PK_DEF)
 Propagates $ x \sim_r c$.
void Gecode::rel (Space *home, const IntVarArgs &x, IntRelType r, int c, IntConLevel icl=ICL_DEF, PropKind pk=PK_DEF)
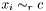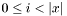 Propagates $ x_i \sim_r c $ for all $0\leq i<|x|$.
void Gecode::rel (Space *home, IntVar x0, IntRelType r, IntVar x1, BoolVar b, IntConLevel icl=ICL_DEF, PropKind pk=PK_DEF)
 Post propagator for 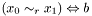$ (x_0 \sim_r x_1)\Leftrightarrow b$.
void Gecode::rel (Space *home, IntVar x, IntRelType r, int c, BoolVar b, IntConLevel icl=ICL_DEF, PropKind pk=PK_DEF)
 Post propagator for 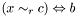$(x \sim_r c)\Leftrightarrow b$.
void Gecode::rel (Space *home, const IntVarArgs &x, IntRelType r, IntConLevel icl=ICL_DEF, PropKind pk=PK_DEF)
 Post propagator for pairwise relation on x.
void Gecode::rel (Space *home, const IntVarArgs &x, IntRelType r, const IntVarArgs &y, IntConLevel icl=ICL_DEF, PropKind pk=PK_DEF)
 Post propagator for relation between x and y.

Function Documentation

void Gecode::rel ( Space *  home,
const IntVarArgs &  x,
IntRelType  r,
IntVar  y,
IntConLevel  icl = ICL_DEF,
PropKind  pk = PK_DEF 
)

Post propagators for $ x_i \sim_r y $ for all $0\leq i<|x|$.

Supports both bounds (icl = ICL_BND) and domain consistency (icl = ICL_DOM, default).

Definition at line 133 of file rel.cc.

void Gecode::rel ( Space *  home,
IntVar  x0,
IntRelType  r,
int  n,
IntConLevel  ,
PropKind   
)

Propagates $ x \sim_r c$.

Definition at line 48 of file rel.cc.

void Gecode::rel ( Space *  home,
const BoolVarArgs &  x,
IntRelType  r,
int  n,
IntConLevel  icl = ICL_DEF,
PropKind  pk = PK_DEF 
)

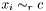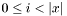Propagates $ x_i \sim_r c $ for all $0\leq i<|x|$.

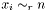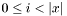Propagates $ x_i \sim_r n $ for all $0\leq i<|x|$.

Throws an exception of type Int::NotZeroOne, if n is neither 0 or 1.

Definition at line 302 of file bool.cc.

void Gecode::rel ( Space *  home,
IntVar  x0,
IntRelType  r,
IntVar  x1,
BoolVar  b,
IntConLevel  icl = ICL_DEF,
PropKind  pk = PK_DEF 
)

Post propagator for $ (x_0 \sim_r x_1)\Leftrightarrow b$.

Supports both bounds (icl = ICL_BND) and domain consistency (icl = ICL_DOM, default).

Definition at line 182 of file rel.cc.

void Gecode::rel ( Space *  home,
IntVar  x,
IntRelType  r,
int  c,
BoolVar  b,
IntConLevel  icl = ICL_DEF,
PropKind  pk = PK_DEF 
)

Post propagator for $(x \sim_r c)\Leftrightarrow b$.

Supports both bounds (icl = ICL_BND) and domain consistency (icl = ICL_DOM, default).

Definition at line 226 of file rel.cc.

void Gecode::rel ( Space *  home,
const IntVarArgs &  x,
IntRelType  r,
IntConLevel  icl = ICL_DEF,
PropKind  pk = PK_DEF 
)

Post propagator for pairwise relation on x.

States that the elements of x are in the following relation:

  • if r = IRT_EQ, then all elements of x must be equal. Supports both bounds (icl = ICL_BND) and domain consistency (icl = ICL_DOM, default).
  • if r = IRT_LE, r = IRT_LQ, r = IRT_GR, or r = IRT_GQ, then the elements of x are ordered with respt to r. Supports domain consistency (icl = ICL_DOM, default).
  • if r = IRT_NQ, then all elements of x must be pairwise distinct (corresponds to the distinct constraint). Supports value (icl = ICL_VAL, default), bounds (icl = ICL_BND), and domain consistency (icl = ICL_DOM). Throws an exception of type Int::ArgumentSame, if x contains the same unassigned variable multiply.

States that the elements of x are in the following relation:

  • if r = IRT_EQ, then all elements of x must be equal.
  • if r = IRT_LE, r = IRT_LQ, r = IRT_GR, or r = IRT_GQ, then the elements of x are ordered with respt to r.
  • if r = IRT_NQ, then all elements of x must be pairwise distinct (corresponds to the distinct constraint).

Definition at line 354 of file bool.cc.

void Gecode::rel ( Space *  home,
const IntVarArgs &  x,
IntRelType  r,
const IntVarArgs &  y,
IntConLevel  icl = ICL_DEF,
PropKind  pk = PK_DEF 
)

Post propagator for relation between x and y.

Note that for the inequality relations this corresponds to the lexical order between x and y.

Supports both bounds (icl = ICL_BND) and domain consistency (icl = ICL_DOM, default).

Throws an exception of type Int::ArgumentSizeMismatch, if x and y are of different size.

Note that for the inequality relations this corresponds to the lexical order between x and y.

Throws an exception of type Int::ArgumentSizeMismatch, if x and y are of different size.

Definition at line 400 of file bool.cc.